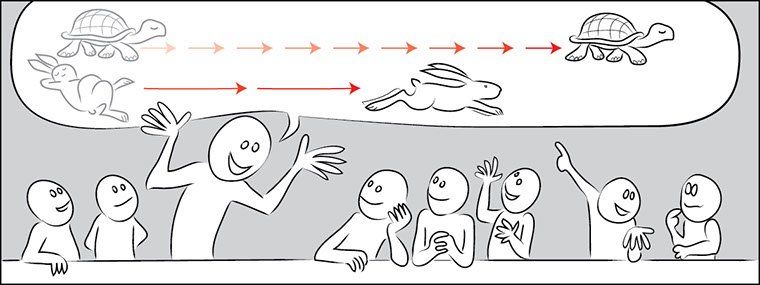
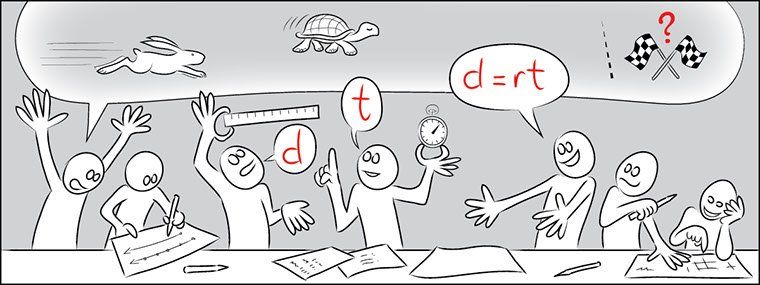
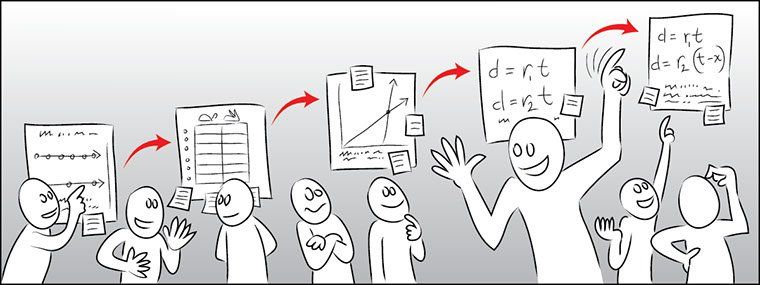
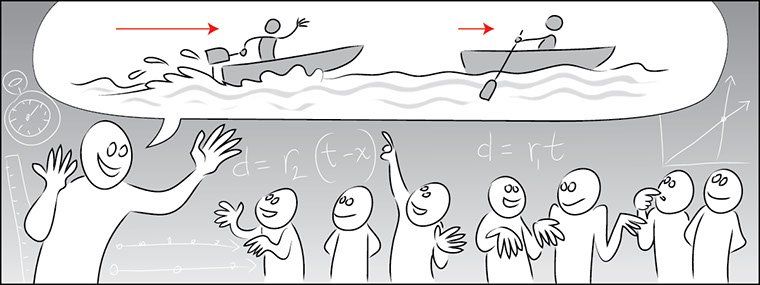
Each Poster Problem has Six Phases:
1. Launch
Teachers set the stage by leading an introductory discussion that orients students to the context of the problem.
2. Pose a Problem
Teachers introduce a mathematical way of thinking about the context and engage students in a preliminary approach that opens the door to the workshop phase.
3. Workshop
The workshop starts with a more challenging and more open-ended extension of the problem. In teams, students plan and produce mathematical posters to communicate their work.
4. Post, Share, Comment
Teams display their posters in the classroom, get to know other teams’ posters, and attach questions/comments by way of small adhesive notes (or similar).
5. Strategic Teacher-Led Discussion
Teachers then compare, contrast, and connect several posters. In the process they highlight a progression from a more basic approach to a more generalizable one.
6. Focus Problem: Same Concept in a New Context
Serving as a check for understanding, this more focused problem gives teachers evidence of student understanding.
Listen to teacher Norm Mattox
talk through the phases.
In a series of videos, mathematics educator Phil Daro
explains the rationale of this approach.
A leading thinker in mathematics education, Professor Alan Schoenfeld
weighs in on sense-making, teacher knowledge, and the Common Core.
Project funding provided by The William and Flora Hewlett Foundation and S.D. Bechtel Jr. Foundation.
This work is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License.